What Are Sine, Cosine, and Tangent?

Do you know what two angles living inside the same right triangle said to each other? The first angle goes, "Hey Thelma (or is it Theta?), I don't mean to go off on a tangent here, but what's your sine?" To which the second angle replies, "Phil (or is it Phi?), I don't know why you even bother to ask, my sine is obviously the same as your cosine!"

Okay, so maybe that's not the best joke in the world, but once you understand sines and cosines, it is kind of funny. Of course, that means that if you don't know the difference between a sine and a cosine, you're currently left out in the metaphorical cold.

Clearly we can't let that happen—and we won't! Because today we're going to learn all about sines, cosines, and tangents.

Recap: trigonometry and triangles

When we talked about the world of trigonometry, we learned that the part of math called trigonometry deals with triangles. And, in particular, it's the part of math that deals with figuring out the relationship between the three sides and the three angles that make up every triangle.

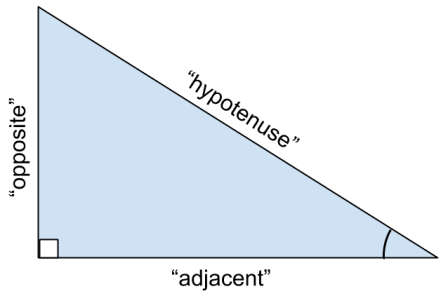
Of particular interest to us is the special type of triangles known as right triangles. Every right triangle has one 90-degree angle (like the corner of a square or rectangle), and two angles that each range between anything larger than 0 degrees and smaller than 90 degrees (with, as we'll talk about in the future, the sum of all 3 angles being 180 degrees).

For our discussion of sine, cosine, and tangent (which, don't worry, are not as complicated as they sound), it's important that we have a way of labeling the sides of right triangles.

As we learned last time, the longest side of a triangle is known as its "hypotenuse." The side opposite the angle we're looking at is known as the "opposite" side (logically). And the side adjacent to the angle we're looking at (the one that isn't the hypotenuse) is known as the "adjacent" side.

Sine, cosine, and tangent

With all of these preliminaries now happily splashing around inside our growing pool of mathematical knowledge, we're finally ready to tackle the meaning of sine, cosine, and tangent. Here's the key idea:

The ratios of the sides of a right triangle are completely determined by its angles.

The ratios of the sides of a right triangle are completely determined by its angles.

In other words, the...

Keep reading on Quick and Dirty Tips